
The square part stands because the most significant power of our variables (x) is two. He says: The vertex of a parabola is a point that represents the extremal value of a quadratic curve.


This standard form to vertex calculator is a free tool to help you with parabolic equations.
Vertex form to standard calculator how to#
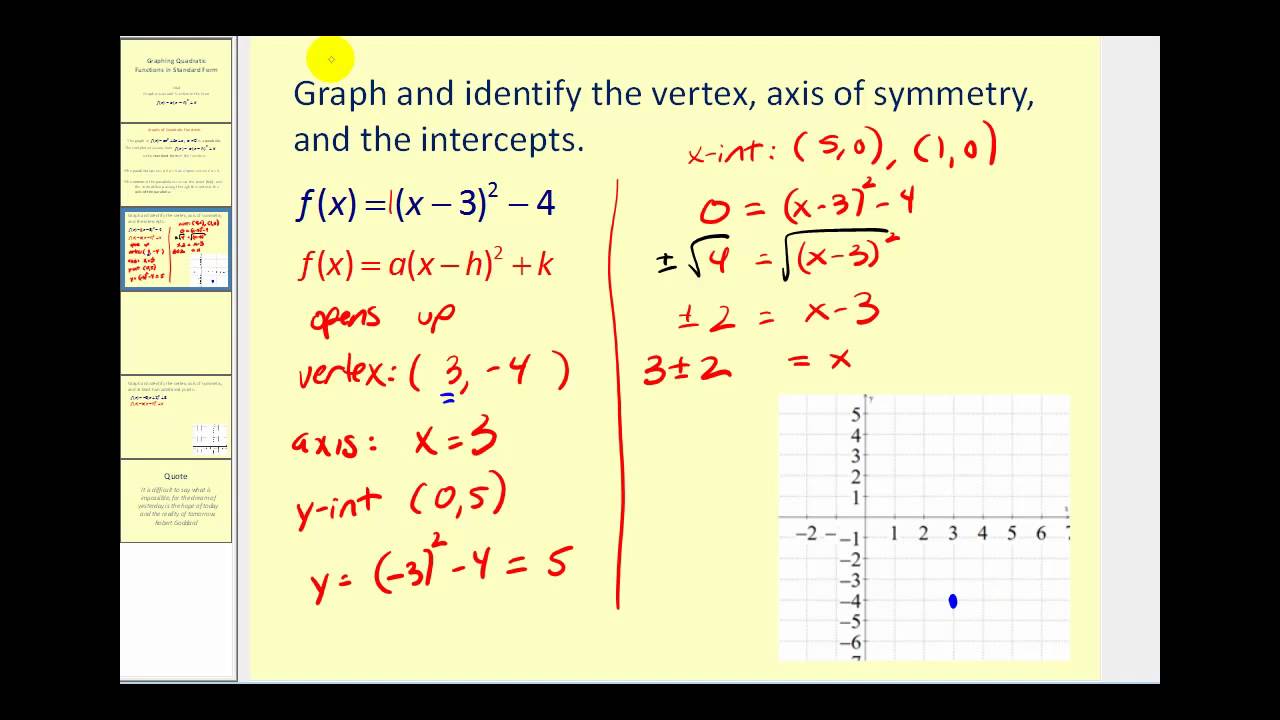
As a result of the comparison, we know how to find the vertex of a parabola: h = -b/(2a) and k = c – b²/(4a). The vertex and standard shape of the parabola: y = 0.25(x + 17)² – 54 and y = 0.25x² + 8.5x + 18.25 Compare the result with the vertex shape of a quadratic equation: y = a*(x-h)² + k For a polynomial $$ p(x) = a_n x^n + a_ = -1 and k=f(-1)=3(-1)^2+6(-1)+4=3-6+4=1 The coordinates of the vertices are (k,h)=(-1,1) You can easily find the vertex from the square equations. The parameter a can never be zero for a vertex form of a parabola (or any other form, strictly speaking). It tells us whether the parabola opens (a > 0) or downwards (a < 0). One of them is one, the same as in the standard form. As you can see, we need to know three parameters to write a square vertex shape. But how do you find the top of a square function? It may be a surprise, but we don`t need to evaluate a square root to do it! In addition, it should be noted that it is possible to draw a square function graph that contains only the parameter a and the vertex. However, if you have a standard shape equation, you can use this calculator to convert it to a vertex shape.
You can find the vertex of a parabola from a vertex equation.


 0 kommentar(er)
0 kommentar(er)
